Notes
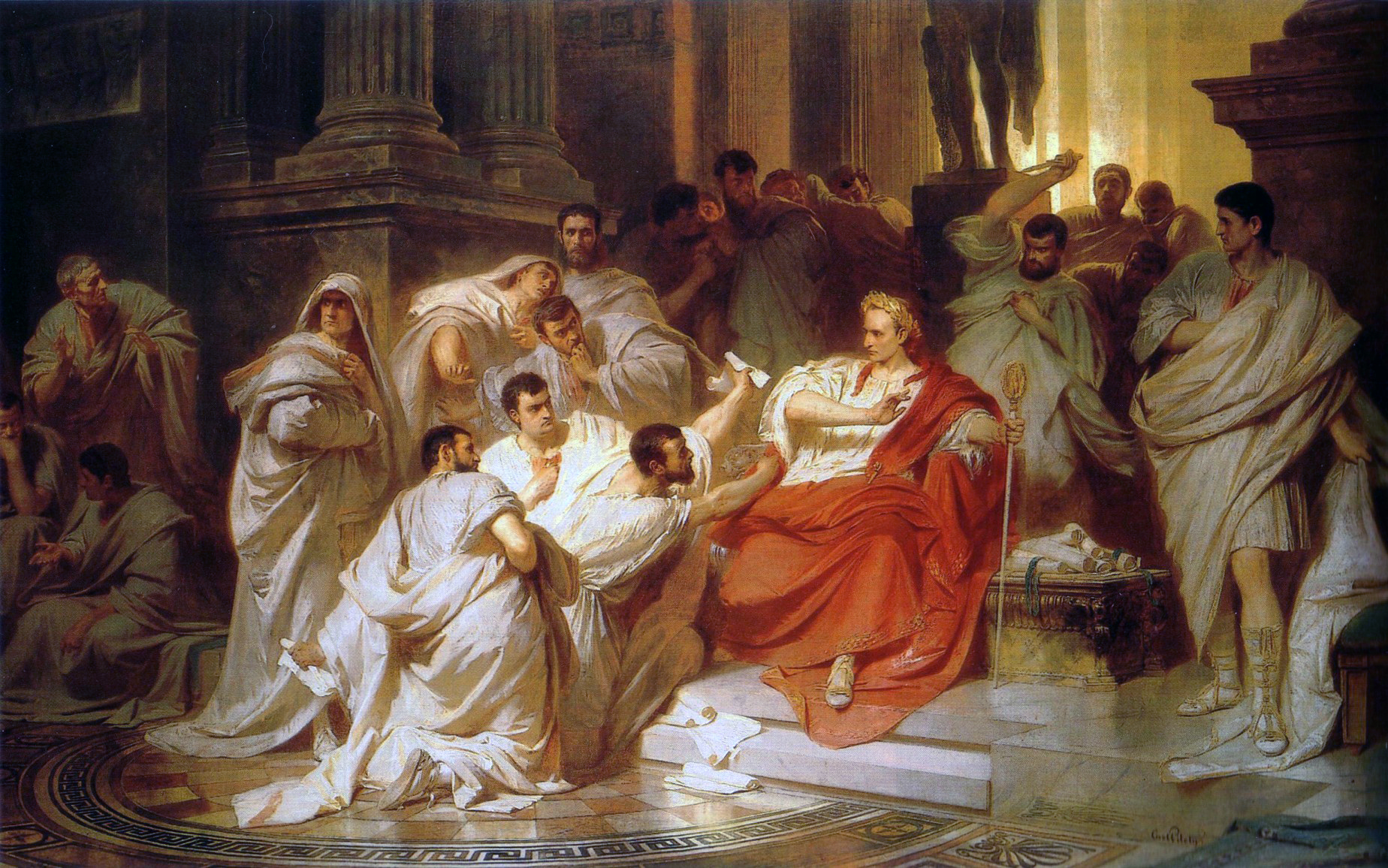
Tolstoy on History
I've been reading War and Peace and one theme that Tolstoy constantly revisits is the great man theory of history vs. the trends and forces theory of history. Time and again, he seems to favour the latter theory. Especially through Prince Andrei's monologues to Pierre before the Battle of Borodino, and then while talking about all those defeats to Napoleon at Austerlitz, and Borodino. The writing is so inspired and I wanted to quote some of the writing here in the hope that you will be encouraged to pick up the book and read it. This is Chapter 28 from Book Three, Part Two of War and Peace.
Many historians say that the French did not win the battle of Borodino because Napoleon had a cold, and that if he had not had a cold the orders he gave before and during the battle would have been still more full of genius and Russia would have been lost and la face du monde eût été changée.
To historians who believe that Russia was shaped by the will of one man—Peter the Great—and that France from a republic became an empire and French armies went to Russia at the will of one man—Napoleon—to say that Russia remained a power because Napoleon had a bad cold on the 24th of August may seem logical and convincing.
If it had depended on Napoleon’s will to fight or not to fight the battle of Borodino, and if this or that other arrangement depended on his will, then evidently a cold affecting the manifestation of his will might have saved Russia, and consequently the valet who omitted to bring Napoleon his waterproof-boots on the 24th would have been the saviour of Russia.
Along that line of thought such a deduction is indubitable, as indubitable as the deduction Voltaire made in jest (without knowing what he was jesting at) when he said that the Massacre of St. Bartholomew was due to Charles IX’s stomach being deranged.
But to men who do not admit that Russia was formed by the will of one man, Peter I, or that the French Empire was formed and the war with Russia begun by the will of one man, Napoleon, that argument seems not merely untrue and irrational, but contrary to all human reality. To the question of what causes historic events, another answer presents itself, namely, that the course of human events is predetermined from on high—depends on the coincidence of the wills of all who take part in the events, and that a Napoleon’s influence on the course of these events is purely external and fictitious.
Strange as at first glance it may seem to suppose that the Massacre of St. Bartholomew was not due to Charles IX’s will, though he gave the order for it and thought it was done as a result of that order; and strange as it may seem to suppose that the slaughter of eighty thousand men at Borodino was not due to Napoleon’s will, though he ordered the commencement and conduct of the battle and thought it was done because he ordered it; strange as these suppositions appear, yet human dignity—which tells me that each of us is, if not more, at least not less a man than the great Napoleon—demands the acceptance of that solution of the question, and historic investigation abundantly confirms it.
At the battle of Borodino Napoleon shot at no one and killed no one. That was all done by the soldiers. Therefore it was not he who killed people.
The French soldiers went to kill and be killed at the battle of Borodino not because of Napoleon’s orders, but by their own volition. The whole army—French, Italian, German, Polish, and Dutch—hungry, ragged, and weary of the campaign, felt at the sight of an army blocking their road to Moscow, that le vin est tiré et qu’il faut le boire.
Had Napoleon then forbidden them to fight the Russians, they would have killed him and have proceeded to fight the Russians because it was inevitable.
When they heard Napoleon’s proclamation offering them, as compensation for mutilation and death, the words of posterity about their having been in the battle before Moscow, they cried ‘Vive l’Empereur!’ just as they had cried ‘Vive l’Empereur!’ at the sight of the portrait of the boy piercing the terrestrial globe with a toy stick, and just as they would have cried ‘Vive l’Empereur!’ at any nonsense that might be told them. There was nothing left for them to do but cry ‘Vive l’Empereur!’ and go to fight in order to get food and rest as conquerors in Moscow. So it was not because of Napoleon’s commands that they killed their fellow men.
Links from the Winter of '24
The Subprime AI Crisis:
"My concern is that I believe
we're in the midst of a subprime AI crisis, where thousands of companies have
integrated generative AI at prices
that are far from stable, and even further from profitable."
How does Goldene Stack?: when does
multilayer Goldene transition into
solid gold?
Optimizing Beer Glass Shapes to Minimize
Heat Transfer: I really
loved reading this paper, so much of it was simply elementary thermodynamics, which
was probably why I could
understand (and appreciate) it. Fascinating!
The bunkbed conjecture is false: this
is a nice example of a
counter-example in graph theory (something that one would think is in the area of
pure mathematics) found via neural
networks. But this is isn't why it is cool, it just happens to be something that
I know about.
The
Complete List
of Trump’s Twitter Insults (2015-2021): This is America.
My
mother texts me instructions to cook silken tofu: a beautiful poem by Sue
Zhao
https://iss-sim.spacex.com/: ISS docking
simulator game by SpaceX, this is
super cool but insanely hard for me.
The Social Radars interview Theranos whistleblower Tyler Schultz: Part
1 and Part
2.
Elle Luna on The Great
Discontent.
Racing's
Deadliest Day, a superb article
on the 1955 Le Mans crash.
Text is the Universal
Interface
Troy, (2004) dir. Wolfgang Peterson
This will be the greatest war the world has ever seen. We need the greatest warrior.
- Nestor to Agamemnon about Achilles.
Achilles: They'll be talking about this war for another thousand years.
Hector: In a thousand years, the dust from our bones will be gone.
Achilles: Yes prince. But our names will remain.
page: Are the stories true? They say your mother was an immortal goddess. They say you can't be killed.
Achilles: I wouldn't be bothering with the shield then, would I?
page: The Thessalonian you're fighting... he's the biggest man I've ever seen. I wouldn't want to fight him.
Achilles: That's why no one will remember your name.
Distilling Stockfish into a Transformer
I recently came across this paper (Grandmaster-Level Chess Without
Search) by Google DeepMind and my interest was sufficiently piqued to
warrant a close read. A first skim gave me the impression that the transformer
learned to play chess by looking at a very large number of board positions + the
corresponding best move. To do this, the researchers fed FEN strings of the boards
before and after the move as input and output respectively to the feed-forward
transformer network. However, the paper does not claim this at all, and instead does
something else entirely, which is to approximate the position-evaluation
capabilities of Stockfish 16 (a
chess engine that evaluates chess positions using tree search) with a feed-forward
transformer network.
It is my opinion that this is not the same as learning how to play chess, but that
is a topic for another day. First of all, I'll just write briefly about what the
paper actually does before adding my thoughts to this. The dataset is prepared by
randomly choosing board positions from games played on LiChess and using Stockfish
to evaluate board positions. Pairs of [positions, moves] are prepared and also
evaluated (about a billion or so of these). Three predictors are trained using this
dataset. The action-value predictor predicts the evaluation given a position and a
move. The state-value predictor predicts the evaluation given a position. The
Behavioral Cloning predictor predicts the best move given a position.
This whole process leads to a predictor that gets very close to approximating SF
behaviour on board states, both seen and unseen. However, because the model does not
use search during inference, it fails in very specific ways. One example discussed
in the paper is blindness to impending three-fold repetition, this happens because
the model has no record of past moves but treats each board position as an
independent sample. The "without search" claim just pertains to the fact that the
model doesn't explicitly search through possible solutions during inference. But a
criticism that I have seen and I agree with is that using SF as an oracle (a fancy
term which means they use it to annotate their dataset) means that we use the
tree-search capabilities of Stockfish directly. And there are cases where the model
requires hard-coding a search in order to finish games. Some details about the game
are hard-coded into the data, for example, the FEN strings (Q: What would happen if
PGN strings were used instead? A: Ask Nicholas Carlini, who created a LiChess bot that
does next move prediction with PGN strings) contain information about
whether castling is allowed.
The only thing the paper overclaims is about Grandmaster level play. The reported
2895 Elo is achieved via online blitz play and Grandmaster norms are given out for
over the board classical chess games. The ELO calculation is also not consistent.
The paper uses BayesElo with anchors as the LiChess ratings (which themselves, are
calculated via the Glicko2 method). Technical details aside, the paper shows games
where the agent misses #3 (checkmate in three moves) because it finds an alternate
#5 mating strategy that ends in five moves. Human GMs don't play this way, and most
beginner endgame exercises are aimed at learning and identifying quick mates. I
think this follows a general trend of engines resorting to really suboptimal play in
poor positions. GMs (or any chess player) don't suddenly drop their level when the
position gets bad.
In conclusion, I liked this paper a lot and I think it is written very well. The
paper doesn't claim that the agent learns the rules of chess, but it is definitely
written in a way that suggests it does. The agent distills the evaluation
capabilities of Stockfish 16, a tree-search based chess engine, into a transformer.
During inference, it outputs an evaluation given a board state. With scale, the
model should only match the capabilities of SF16, and I don't see why it would be
better than SF in any way. Practically, I don't see it deployed anywhere for
analysis purposes considering that inference would need GPUs and there wont be any
significant new ideas.
One implementation detail that I did not understand initially was the use of value
binning. Even though the predictors output a real number (winning percentage), the
range (0-100%) is divided uniformly into $K$ bins, with $K$ being a design
hyperparameter. This is done only for the action-value and state-value predictors.
But I have a theory that value binning is done simply because log-loss works better
while training transformer networks even though an MSE feels more intuitive. Another
theory is that it is better to bin because a 97% winning percentage is not that
different from a 90% winning percentage when it comes to chess. Both positions would
be annotated as "completely winning" by an expert chess player.
Anyway, I have spent a lot more time on this than I originally planned to, but I
think there is a lot of benefit from reading papers like these, a lot of the heavy
lifting behind some of these results is just really good and inspired design choices
and after a point, you need to be good at noticing these details from papers rather
than just the transformer and the losses. End of discussion. Write to me if you want
an annotated copy of the paper.
New+Mersenne+Largest Prime Found
A new prime has been found! This rumour was floating around for a few days now on
the mersenne.org forums but all tests are complete and they have decided to announce
it. It is a gigantic number. $$2^{136279841} - 1.$$ There is a collaborative project
called the Great Internet Mersenne Prime Search where people all over the world
contribute computational resources to find more prime numbers. The details are in
the press release and will no doubt be picked up by most media outlets + pop-science
media pretty soon so I wont do too much exposition and waste my time. I will,
however, write why this is exciting to me, and should be to anyone that likes
science.
When the last Mersenne prime was discovered (these primes are easier to search for
and easier to test for using the Lucas-Lehmer test), I was really into Number Theory
and my friends and I really put a lot of thought into how one would find the next
Mersenne Prime. To give some context, three Mersenne Primes were found in three
years making it seem, to the amateur mathematicians in us, that this was not too
unachievable. As it turned out, it took almost six years for the next one to be
discovered. There are a few things that have changed since then, first is that they
use a probabilistic search to filter out non-primes quicker, and secondly, this is
the first time GPUs were involved in this project. Usually, these initial
breakthroughs in science are followed by a string of other results improving on
these methods. So, we might get a few more primes in the next two or three years. I
wonder how the Nvidia stock price will react to this information.
India win everything at the Olympiads!
Gold in the Open
section with two rounds to spare. Gold in the Women
section. Board
3 gold for Divya with a TPR of 2608. Board 4 gold for Vantika with a TPR of 2558.
Board 1 gold for Gukesh with a historic TPR of 3056 (second on the all time TPR
list). Board 3 gold for Arjun with a TPR of 2968. The Gaprindashvili Cup for best
combined performance. Third place and fifth places respectively for the Arjun and
Gukesh in the live rating charts, and as a bonus, Vishy snuck back into the top 10
:)




Links from the Monsoon of '24
Vettel's eyes, they
never lie :)
Haemoglobin-based
Oxygen Carriers: a substitute for blood?
The Talk, smbc comics, if
you don't talk to your kids
about quantum computing, someone else will.
The
Times' Profile on Hannah Neeleman:
yikes!
Jean-Pierre Serre is still doing math
research, he's 98 now!
Mixed-input
matrix multiplication performance optimizations, this is such a nice blog
post from Google Research that
discusses ways to perform efficient mixed-input (S8 and F16) matrix multiplication.
Very reminiscent of the old
Google.
Marina Abramovic anecdotes are all wonderful, what an artist: The
bittersweet story of Marina Abramović's epic walk on the Great Wall of
China
Not only is this paper completely unhinged, but they have a wonderful line in it,
"Because this paper was
written in 2024, we include an obligatory section involving generative AI and
LLMs". An Abundance of Katherines:
The Game Theory of Baby Naming
Sabalenka's
topspin stats at the US Open 2024: super interesting reddit thread about the
technical differences between
men's and women's tennis.
Grammar-checking
Taylor Swift
The
FBI files on Paul Erdos
An Elliptic Curve with Rank >= 29 has been found!
The elliptic curveThis Reddit thread from r/math is very interesting. Elliptic curves are super-important in number theory. Many unsolved problems depend on our understanding of elliptic curves. I remember reading all I could about Fermat's Last Theorem in 2021 and learning the basics of elliptic curves so I could get a rudimentary understanding of the Taniyama-Shimura conjecture. Most elliptic curves have ranks of 0 or 1, so finding curves with higher ranks is very hard. I mean, look at the coefficients!
y² + xy = x³ - 27006183241630922218434652145297453784768054621836357954737385x + 55258058551342376475736699591118191821521067032535079608372404779149413277716173425636721497
has rank at least 29.
https://web.math.pmf.unizg.hr/~duje/tors/rk29.html
- robinhouston (@robinhouston) August 30, 2024
on the sums of multiples of two numbers
I came across a strange pattern when computing the sum of multiples of 2 and 3 below
1000, below 10000 et cetera. The sum of multiples of 2 and 3 less than
increasing powers of 10 are as follows, $\{32, 3317, 333167, 33331667, 3333316667,
\dots\}.$ This intrigued me and I wanted to check if this behaviour held for other
primes as well and found that it does for a bunch of others. Examples: $f(2,5)=\{25,
2950, 299500, 29995000, 2999950000,\dots\},$ and $f(3,5)=\{23, 2318, 233168,
23331668, 2333316668,\dots\}.$
def sumMul(p1: int, p2: int, b: int, n: int) -> int:
A = (b ** n - 1) // p1
B = (b ** n - 1) // p2
C = (b ** n - 1) // (p1 * p2)
print(A, B, C)
return p1 * A * (A + 1) / 2 + p2 * B * (B + 1) / 2 - (p1 * p2) * C * (C + 1) / 2
res = []
for i in range(1, 9):
res.append(sumMul35(3, 5, 10, i)) # change this line
print(res)$x^n/n!$ and Stirling's formula
I saw this nice problem on Mathstodon a few months ago and decided to take a look today. It turns out that the curves of $x^n/n!$ have very similar shapes but shifted. What is the size of the shift between the curves?

The easiest way to do it is by using Stirling approximations, on which I did some rudimentary speed analysis sometime before. Assume that the value we're trying to find the spacing at is $t.$ Then we need to find the corresponding value of $x$ for two successive curves. $x^n / n! = t.$ Recall Stirling's formula and substitute it into this: $$n! \approx (n/e)^n \sqrt{2\pi n}$$ $$x^n / (n/e)^n \sqrt{2\pi n} = t$$ Two things happen here when $n$ is large and $t$ is small, we can ignore the effects of $\sqrt{n}$ in the product with $n^{n}$ firstly and notice that $t^{1/n}$ equals one. So we get $$x = n/e$$ and the resulting spacing is simply $1/e.$ I thought this was very neat, and a good example of street-fighting calculations that are seemingly too complex. I would say that the hardest part in this entire thing was plotting the graphs, they needed some programming acrobatics to get the axes right.
def comp(n: int) -> list:
x = []
y = []
for i in np.linspace(0, 10, 1000):
if ((i ** n) / math.factorial(n)) <= 2:
x.append(i)
y.append((i ** n) / math.factorial(n))
return x, y
for i in range(2, 20):
plt.plot(comp(i)[0], comp(i)[1])
plt.xlabel("x")
plt.ylabel("x^n/n!")
plt.show()Olympic Medals per Capita
Olympics Medals per Capita measures the number of Olympic medals won by a country relative to its population. They also have a weighted measure, and another metric relative to the GDP of the country. All of these metrics are better than raw medal tally. They have a description in their 'about' page which goes:
As you scan the Olympic medal tally, one thing stands out: larger countries tend to win more medals. There are a few notable exceptions, though, including New Zealand, which wins many more medals than you'd expect of a country its size.
However, they are very kind to India, which is definitely an exception, seeing how we regularly have nothing to show for our booming population. We need to get an award for transcending the law of averages by not even accidentally producing a good bunch of Olympic athletes over the last thirty odd years.
[wip] Thoughts on Novak Djokovic
There is a very strong chance no one will have his resume ever again. 24 slams and
counting, 7 year-end championships, and now the Olympic Gold. History was made at
Philippe Chatrier, an unlikely place for Novak to do it, considering that there was
another man for whom this court has been a kiddie playground for almost twenty
years. Novak Djokovic has always been an interesting player to me, he's
definitely the greatest, but it feels like he needs to do something more than win
for him to be in the debate with Federer and Rafa.
I remember reading an article about Rafael Nadal sometime ago where the writer was
talking about how Rafa was perpetually in second place all his career (apart from in
Roland Garros, and a couple of years here and there). First to Roger Federer and
then to Novak Djokovic. The writer wondered how it must feel to always be the person
that comes up short. How it must feel to always die at the end of the book.
With Novak, it is quite a different question. He has always been second favourite,
first to the Roger-Rafa fans and now with the Sinner-Alcaraz fans. He is continually
the anti-hero for the crowds. How must it feel when everyone is rooting for you to
die at the end of the book?
Maybe that is why he fights so hard. Maybe it is when his back is against the wall,
that he becomes Novak Djokovic.
Colonization of Venus
I came upon this delightfully technical article on the possibilities of a Venus
colony, wait for it, not on the surface of the planet but in the atmosphere of
Venus! around 50 km above the surface. The rationale is that while the surface is
very hostile to human life, the zone 50 km above is the most earth-like in the solar
system. I did not know this, and I wonder how they ascertained that. First
principles wise, space is hard. There's the minor issue of oxygen, but we also need
carbon, hydrogen, nitrogen, and basically every element that we have evolved to live
in harmony with on earth. Apart from all that, winning the under-appreciation
contest, is gravity. We're going to go all loopy, literally and figuratively, if we
don't have gravity.
Long story short, the surface of Venus sucks. Surface temperature of 737 K (300 K on
earth), surface pressure of 9.2 MPa (0.1 MPa on earth) and an atmosphere with 96.5%
CO$_2$ which, get this, exists in a supercritical state at the surface because of
the pressures. The worst part, there's no sun, because of all the poisonous cloud
cover.*
The proposal is this. We colonize the air of Venus. We build floating cities, much like vimanas or the golden floating city of Tripura. Considerations include breathing (oxygen supply), solar radiation shielding, anti-corrosive measures in the air, pressure differentials and repairs in case of gas leaks, length of the solar day (116.8 times that of earth) and resources (both for maintenance and exploration purposes)
Space is wonderful and it is probably my fear of getting nerd-sniped into eternity that I don't spend more time on these ideas.
References:
1. Venus' atmosphere: Composition, clouds and weather on Space
2. Constraints on a potential aerial biosphere on Venus by Dartnell et al, in Icarus
3. Colonization of Venus by Geoffrey Landis
Like a river of steel it flowed, grey and ghostlike.
The entrance of the German army into Brussels has lost the human quality. It was lost as soon as the three soldiers who led the army bicycled into the Boulevard du Régent and asked the way to the Gare du Nord. When they passed, the human note passed with them. What came after them, and 24 hours later is still coming, is not men marching, but a force of nature like a tidal wave, an avalanche or a river flooding its banks. At this minute it is rolling through Brussels as the swollen waters of the Conemaugh Valley swept through Johnstown.
At the sight of the first few regiments of the enemy we were thrilled with interest. After they had passed for three hours in one unbroken steel-grey column we were bored. But when hour after hour passed and there was no halt, no breathing time, no open spaces in the ranks, the thing became uncanny, inhuman. You returned to watch it, fascinated. It held the mystery and menace of fog rolling toward you across the sea.
The grey of the uniforms worn by both officers and men helped this air of mystery. Only the sharpest eye could detect among the thousands that passed the slightest difference. All moved under a cloak of invisibility. Only after the most numerous and severe tests, with all materials and combinations of colour that give forth no colour, could this grey have been discovered. That it was selected to clothe and disguise the German when he fights is typical of the German Staff in striving for efficiency to leave nothing to chance, to neglect no detail.
After you have seen this service uniform under conditions entirely opposite you are convinced that for the German soldier it is his strongest weapon. Even the most expert marksman cannot hit the target unless he can see. It is a grey-green, not the blue-grey of our Confederates. It is the grey of the hour just before daybreak, the grey of unpolished steel, of mist among green trees...
This army has been on active service three weeks, and so far there is not apparently a chin-strap or a horseshoe missing. It came in with the smoke pouring from cookstoves on wheels, and in an hour had set up post office wagons, from which mounted messengers galloped along the line of column distributing letters and at which soldiers posted picture postcards.
The infantry came in files of five, 200 men to each company; the lancers in columns of four, with not a pennant missing. The quick-firing guns and field pieces were one hour at a time in passing, each gun with its caisson and ammunition wagon taking 20 seconds in which to pass.
The men of the infantry sang “Fatherland, My Fatherland”. Between each line of song they took three steps. At times 2,000 men were singing together in absolute rhythm and beat. When the melody gave way, the silence was broken only by the stamp of ironshod boots, and then again the song rose. When the singing ceased, the bands played marches. They were followed by the rumbles of siege guns, the creaking of wheels and of chains clanking against the cobble-stones and the sharp bell-like voices of the bugles.
For seven hours the army passed in such solid column that not once might a taxi-cab or trolley car pass through the city. Like a river of steel it flowed, grey and ghostlike. Then, as dusk came and a thousands of horses’ hoofs and thousands of iron boots continued to tramp forward, they struck tiny sparks from the stones, but the horses and men who beat out the sparks were invisible.
At midnight, pack wagons and siege guns were still passing. At seven this morning I was awakened by the tramp of men and bands playing jauntily. Whether they marched all day or not I do not know; but for 26 hours the grey army rumbled with the mystery of fog and the pertinacity of a steamroller.
- Richard Harding Davis
Links from the Summer of '24
Millikan's
Oil Drop Experiment is flawed (?)
Illuminating (and sometimes funny) discussion on Russians
and voting
Nice video about the XOR perceptron problem: Sisyphus
Perceptron
I think Elle Cordova is just
wonderfully clever. I love watching
her videos!
JavaScript Bloat in 2024, if I could
have math typesetting without JS,
I'd get rid of JavaScript entirely
I learnt a lot about potato botany from this question on potato
evolution in The Martian
A Tour of Johannes
Vermeer narrated by Stephen Fry.
Generate random mathematics
papers
David
Hume, the Buddha, and a search for the Eastern roots of the Western
Enlightenment by Alison Gopnik
No
Regrets: Boris Spassky at 60:
a fascinating interview from the 10th World Champion
Why do doctors still use
pagers?: a good example of
communicative friction being a good thing
Karpov, Kramnik, Anand vs.
Karjakin, Nepo, Dubov
Gene
therapy reverses hearing loss: we are on the brink of a great golden age in
medical science
Being
13: Social media is the worst thing that has happened to teenage girls
Geometry Puzzle
I found a nice puzzle on Twitter that I want to add some thoughts about here. The
problem is that you need to find the shortest route from A to B by touching the
perimetre of the circle on the way. We can solve this analytically, by considering a
parametric form of the circle and minimizing the distance from the two points but we
would ideally require a ruler and compass construction of the solution. Apparently,
this problem can be traced way back to Ptolemy in 150 AD.
References:
1. Alhazen's
Billiard Problem on Wolfram Mathworld
2. Circular
Billiard by Drexler and Gardner
3. Twitter
thread

There are three special cases that I want to note here:
1. If B=A, then the shortest route is the round trip from A to the nearest point on
the circle.
2. If B or A is on the perimeter, then the shortest route is the straight line from
A to B.
3. If B or A is on the center of the circle, then the shortest route is along the
radius of the circle.
Vielen Dank für alles, Herr deutscher Scharfschütze
Toni getting emotional after the second goal against Bayern was the clearest of the
signs. In the ten years I've seen him play, the man has never let his mask down. Up
by four goals or down by two, he remained the eye of the storm, a calm and unruffled
presence in the center of the pitch. Turn on a Real Madrid game, and in the centre
of the pitch is The German Sniper, standing over the ball, his eyes piercing the
opposition defence, tracing the path that the ball will soon follow.
Never has a player of this calibre, since Zidane, retired at the peak of his career.
Toni goes out at the top, retiring from a starting position at the greatest club in
the world. He kept his promise to himself, to retire at Madrid. No one expected
this, all eyes were on Luka and all along, it turned out to be Toni that hung up
first. It's been like this at Real Madrid for a few years now. It started with
Cristiano leaving, and Toni is gone now. I watched them all play football, I am the
lucky one.
Like Luka Modric wrote, there won't be another Toni Kroos. Toni Kroos was the
orchestrator of victories, he controlled the Madrid midfield for a decade, holding
the center of the squad, usually as the last man. The greatest deep-lying playmaker
of all time. I remember so much about this man, his millimeter-precision passes, the
trademark Kroos feint while he receives the ball, his diagonal switches to Carva.
Teams these days switch play by passing one man to another, and I often wonder why
they're so slow, but they don't have a Toni Kroos to play that pin-point diagonal
ping. No one presses the Real Madrid backline for this one reason, because all Real
need to do is get the ball to Toni, and he's going to send it to the wing, and we
score within a handful of touches. There were midfielders that were stronger than
him, or faster than him, or far more skillful. But he beat all of them because he
could outthink them.
In his 10 years at Real Madrid, he had pass completion percentages of 92%, 92%, 94%,
92%, 93%, 93%, 94%, 94%, 95%, 95%
with an average of around 2100 passes per season. Do you know what means? Toni Kroos
misses around 100 passes, over the
entire league season! I will let you read that again. Remember that he plays in Real
Madrid, a team that loves to score
goals, and he has to make a large percentage of those passes in attacking
transitions. He is unrepeatable, I hope he
wins the 6th UCL, then wins the Euros at home, and gets that Ballon D'Or that he
deserves so much for the career he's
had.
Remember a ball skimming along the grass into the goal. Remember a pass squeezing
between the gloves of the keeper and
the far post. Remember a goal scored from the corner spot. Remember a vertical pass
beating three lines of defenders.
Remember a feint in the penalty box to shake off the press. Remember the ball
fizzing in the air to the right back's
feet. Remember the white shoes with the blue tongue and heel. Remember the ice-cold
composure on and off the pitch. Remember the personal integrity upheld all through
the fame and the success. Remember Real Madrid's
greatest number eight. Remember
Toni Kroos.




Sebastian Vettel Drives Senna's McLaren Mp4/8 in Imola
Seb drove Ayrton Senna's McLaren MP4/8 in Imola before the race last night. What a magnificent beast that car is, the wail of the naturally aspirated V8 strikes straight into the heart. The gunshot sounds while the car downshifts are beautiful. Seb is probably the best driver to do this stuff, not only does he have tremendous respect for the history of the sport and its legends, he genuinely loves racing in those cars. He was properly throwing the car into every corner around the circuit. But the best part was when he brought out the Brazilian and Austrian (for Roland Ratzenberger) flags. He held out the flags, and proceeded to drive two laps steering with one hand. He was just twenty seconds off the pace too!! He talks about how it felt in the cockpit and why he got out the flags in the interview just after he did donuts on the main straight and gave 2013 Indian GP flashbacks by bowing to the car.

The race itself was a slow-burner, Max looked very comfortable until the final fifteen laps when he had to hold off a very rapid Lando Norris. That McLaren really came to life in the second stint. Both Oscar and Lando were extremely quick. A couple more laps and Lando would've had two race wins in a row.
International Women in Mathematics Day!
Happy Birthday to Maryam Mirzakhani, mathematician, and Fields Medallist.
One of my greatest inspirations. Watching Secrets of the Surface reminded me why
I loved mathematics so much. Her story will live forever.
The Games of Robert James Fischer
I've resumed my chess practice after months of disinterest and I'm going about it by playing classical games and analyzing former world champion games to pick up a few things here and there. I'm starting with Bobby Fischer and my references are two books: My 60 Memorable Games by the great man himself, and Russians versus Fischer, which I think I first heard about in an interview by five time world champion Viswanathan Anand and has stuck with me ever since.
Recreational Math Dump
Some fun questions I've been trying to solve on and off this month.
* The Fibonacci numbers count the number of ways a natural number can be expressed as the sum of 1s and 2s while considering the order. What is the average number of 1s and 2s needed to create a sum equal to any natural number? Ref: an average number of 1s and 2s by Joshua Bowman.
* Choose random numbers between 0 and 1, uniformly and independently, until the sum of the numbers exceeds 1. What is the expected number of terms?
* Let $f:\mathbb{R}\rightarrow\mathbb{R}$ be a continuous function such that for all real numbers $x$ and $y,$ $$f(x+y)-f(x)-f(y)=xy+x^2y+xy^2$$ and $f(6)=69.$ Find $f(2\sqrt{6})$.
Liquefying Silica
So much of the heavy lifting in the previous note about topographical heights involved calculating the liquefying energy needed for a silica molecule. I wanted to expand on that a bit in this note so I can provide myself closure about this topic that consumed me for a few hours.
All depends on the structure of the silica molecule. In the crystalline form, the SiO$_2$ lattice has each O atom bonded to two silicons and each Si atom bonded to four oxygens. For simplicity, we can consider this configuration to have zero entropy ($S=0$). In a liquid state, we can think of some fraction $(f_N)$ of the total molecules ($N$) being "disordered" by moving into holes, and then the entropy will be $S=k_B\ln{N\choose f_N}$. Another simplification ensues after we assume the liquid to be in the highest disordered state possible and the change in entropy per molecule will be $\Delta s\sim k_B\ln{2}$.
There are three steps in the process now, first we need to detach single SiO$_2$ molecules from the lattice, but to do that, we need to break bonds, or more accurately, overcome the binding energy of the bonds. Finally, we need to ask whether it is actually advantageous to completely detach every silica molecule from the lattice.
Each oxygen atom is bounded to one silicon atom in the molecule, but in the lattice, it is bounded to four silicon atoms. So we first need to break $3/4$ bonds. But each oxygen atom is part of two molecules, and so we're double counting. Therefore, we only need to break $3/8$ bonds.
Next, from high school physics, we remember that breaking a bond at the Bohr radius of $0.5$ Å is 13.6 eV. Silicon is on the second row of the periodic table and so we can assume a linear addition of an angstrom. And because two atoms form a bond, the binding energy of a single bond is $$E_{b}\sim Ry\cdot\frac{0.5\text{ Å}}{2\times1.5\text{ Å}}\sim2\text{ eV}.$$ But do we really want the binding energy? Breaking the lattice would mean converting the crystal to a gas, but we only want to achieve liquefaction. Assume that bond length changes by some $p$ % where $p<5$. Then we get the new binding energy $$E_{b}\sim Ry\cdot\frac{0.5\text{ Å}}{2\times1.5\text{ Å}}\cdot\left(\frac{0.0p}{1.0p}\right)\sim0.1\text{ eV}.$$
Isostasy and Mountain Heights
Earth's mantle is mostly silicate rock and behaves as a viscous fluid in geological time (think large time scales). Oceanic crust and continental crust is primarily formed by partial melting of the mantle. There's a gravitational equilibrium between the crust and the mantle and different topographical heights exist on earth because of this static equilibrium. This equilibrium depends on the thickness and density of the crust.
Apparently, the Himalayas are not in isostatic equilibrium (which is a fancy way to say that they're still rising) and mountains primarily rise that high because they're low density. Think of this as them being huge, but having less mass, so they don't crumble. One important point we'll come back to is that mountains fail at their bases. When a mountain gets too high, they suffer plastic deformation at the base.
There are two major theories of isostasy. One that treats the lithosphere (the crust basically, but the more you read about it, the more you want to use the scientifically precise term) as a sheet floating on the mantle with different densities at different heights. This theory straight up applies Pascal's Law (hydrostatic pressure is the same at the same elevation for a fluid in static equilibrium) to all points in the crust. The second treats the lithosphere as an elastic plate that bends and thereby distributes loads.
If the maximum height a mountain can reach is $H$, this is the height at which the energy lost as the mountain "falls" equals the energy needed to cause plastic deformation at the base. Doing the computations for each molecule, we have $$mgh=E_p,$$ where $m=AM$, the atomic mass units in the molecule, $h=H$, the maximum height before deformation, and $E_p$ being the energy needed to cause plastic deformation. Note that Weisskopf uses $E_1$ for this. To cause plastic deformation, we need to liquefy the molecule without breaking the atomic structure. This will require a small fraction ($\epsilon$) of the binding energy $B=\gamma Ry$ where $Ry$ is the Rydberg unit. If we assume that the mountain is a block of SiO$_2$, the atomic mass will be 60 amu. And so the maximum height will be $$H=\epsilon\frac{\gamma Ry}{AMg}\sim \boxed{10\text{ km}}.$$ Later in the paper referenced below, Weisskopf shows a wonderful argument about the maximum height ($H$) of a topological structure on a planet being inversely proportional to the third root of the number of nucleons ($N_p$) in the planet. $$H\propto\frac{1}{N_p^{1/3}}.$$
Update: it turned out that this nucleon argument is neither as fancy or convuluted as I first thought it would be. Rahul Goel pointed out that for any object, $V\times\rho=m$ and mass is just the number of nucleons in an object, and volume is length cubed. And therein lies the intuition for the above equation. Other questions that were raised in the discussion were 1) How do you know that plastic deformation is what occurs when mountains fail at their bases and 2) Is it true that huge mountains usually grow isometrically?
References: Mantle (geology) | wikipedia.org, Isostasy | wikipedia.org, Of Atoms, Mountains, and Stars: A Study in Qualitative Physics | science.org by Weisskopf.
The Tortured Poets Department is out!!
The Tortured Poets Department. An anthology of new works that reflect events, opinions and sentiments from a fleeting and fatalistic moment in time - one that was both sensational and sorrowful in equal measure. This period of the author’s life is now over, the chapter closed and… pic.twitter.com/41OObGyJDW
— Taylor Swift (@taylorswift13) April 19, 2024
It’s a 2am surprise: The Tortured Poets Department is a secret DOUBLE album. ✌️ I’d written so much tortured poetry in the past 2 years and wanted to share it all with you, so here’s the second installment of TTPD: The Anthology. 15 extra songs. And now the story isn’t mine… pic.twitter.com/y8pyDK8VTd
— Taylor Swift (@taylorswift13) April 19, 2024
2023 Turing Award
Avi Wigderson has won the 2023 Turing
Award "for foundational contributions to the theory of computation,
including reshaping our understanding of the role of randomness in computation and
mathematics, and for his decades of intellectual leadership in theoretical computer
science"
Avi also won the 2021 Abel Prize! Making him the first person to hold the double (me
when?). Probability is having its moment in the sun this spring.
Temperature, and some more thermodynamics
Thermometers wouldn't work if energy didn't spontaneously flow from two objects in contact with each other, and temperature is objectively, just something you measure with a thermometer. (of course, we're talking about traditional mercury thermometers here, and not stuff like infrared thermometers)
One definition for the average kinetic energy of an ideal gas (with three degrees of freedom) is $$\bar K=\frac{3}{2}k_BT$$ and we can get a bad definition of temperature by moving the terms around. Note that we got this equation from the equipartition theorem which states the average energy of a system in which individual elements have $n$ degrees of freedom is $\displaystyle\frac{1}{2}nk_BT$.
Fundamental Assumption of Statistical Mechanics: All accessible microstates are equally likely for a system in thermodynamic equilibrium.
Entropy is a statistical measure of how many possible arrangements exist for a system. Define multiplicity $\Omega$ for an Einstein Solid with $N$ quantum harmonic oscillators and $q$ units of energy as $$\Omega={q+N-1\choose q},$$ and energy units will flow in such a way as to maximize the product of individual multiplicities of the objects in contact with each other. Concretely, there are systems $A,B$ with $N_A,N_B$ oscillators and $q_A,q_B$ units of energy in contact with each other. They have individual multiplicities of $\Omega_A,\Omega_B$ and the units of energy $q_A,q_B$ will flow in a way that maximizes $\Omega=\Omega_A\cdot\Omega_B.$ The constraint obviously being that $q_A+q_B$ is always fixed. The graph of $\Omega$ versus $q_A$ looks like a Dirac Delta function with a very narrow peak near the middle, which is intuitive (if you accept that multiplicities should be maximized, because the $\displaystyle{n\choose k}$ function has it's maximum at $2k=n$)
Now, entropy is just the natural log of this multiplicity and has units [energy]$\times$[temperature]$^{-1}$.$$S=k_B\ln{\Omega}$$ The Second Law of Thermodynamics: (1) The entropy of an isolated system tends to increase. (2) A system in thermodynamic equilibrium is most likely to be found in the macrostate (configuration of $q_A$ and $q_B$ for example above) of highest entropy.
Finally, temperature is defined as $$\frac{1}{T}=\frac{\Delta S}{\Delta E}.$$ Which is why temperature is a measure of the tendency of an object to give up it's energy to another object it comes in contact with. Reference/Inspiration: Temperature as Joules per Bit
Infinitesimal Worms
I've always loved the joke where you bite into an apple and find a worm but then you
have to be relieved about it because that is still better than biting into an apple
and finding half a worm. Extending this further, you would prefer half a worm in
your apple than a quarter of a worm. So on and so forth, until it means that the
worst case scenario is when you don't find any worms in your apple.
What is worse than finding $1/n$ of a worm in your apple? Finding $1/n^2$ of a worm
in your apple. Hilariously, this works for cases where $n\in\mathbb{R}^+$ except
that the grammar doesn't hold up. Example: for $n=1/7,$ what is worse
than finding 7 worms in your apple? Finding 49 worms in your apple.
2024 Abel Prize
Michel Talagrand has won the 2024 Abel Prize "for
his groundbreaking contributions to
probability theory and functional analysis, with outstanding applications in
mathematical physics and statistics."
- The Law of Large numbers and Talagrand's inequalities
- Stochastic Processes
- Concentration of measure: The sum of a large number of sufficiently independent
random variables will be concentrated in an interval which is much narrower than is
intuitive. (Think of the curse of dimensionality)
The citation had callbacks to applications of Talagrand's work in Large Language
Models and I'm wondering how much of that was a publicity stunt by the Abel Prize
committee. I'm sure there are far more exciting applications and that the inclusion
of LLMs at a time when their hype is at its peak is just a coincidence.
Links from the Spring of '24
Every Author as First Author
Problems
with grading students on a curve
rotation with three shears
Roger Federer's Neo Backhand in the
2017 Australian Open
52 cards win a
dollar: You have 52 playing cards (26
red, 26 black). You draw cards one by one. A red card pays you a dollar. A black one
fines you a dollar. You can stop any time you want. Cards are not returned to the
deck after being drawn. What is the optimal stopping rule in terms of maximizing
expected payoff?
Songs
with
parenthesis in the titles: or another analysis where Taylor Swift shows up
as a statistical anomaly.
Thermodynamic Linear Algebra:
Harmonic Oscillators ftw
Intelligent
Machinery: Intelligence is a search problem
1012 and other
such numbers
AOH1996: something to watch out
for, Phase 1 Clinical Trials have begun
Richard Hamming on Claude Shannon
He wants to create a method of coding, but he doesn't know what to do so he makes a random code. Then he is stuck. And then he asks the impossible question, 'What would the average random code do?' He then proves that the average code is arbitrarily good, and that therefore there must be at least one good code.
Who but a man of infinite courage could have dared to think those thoughts?
[wip] Dimensional Analysis on Loss Functions
One of my favourite tricks to minimize errors while doing high school physics was to use dimensional analysis as a sanity check on all my equations. It is easy to see that gradient descent is not dimensionally sound, and so I have some thoughts on whether we can reinvent concepts like loss functions using dimensional analysis of gradient descent. For example, if we're trying to minimize the loss on a physical quantity like length, what dimensions would the gradients be, and what dimensions would the learning rates be. What happens to dimensions in neural networks. This is something for me to think about and update later. Watch this space!
Silman's Complete Endgame Course
A few days ago I got my hands on a copy of Silman's Complete Endgame Course, and I'm finally starting to dig into it. I think I'm going to learn a lot from it. The only endgame book I have really studied before was a slim book by Averbakh called Chess Endgames: Essential Knowledge, and I really do not think that Averbakh's book takes you through to master level the way that Silman promises to. I decided to jump into Silman's book at the class “A” level, because it looked as if I knew everything in the chapters up to class “B,” but already in the class “A” chapter there were some things I didn't know.
The class “A” chapter starts out with rook and rook-pawn versus rook positions, and already it has some great stuff. How many open files do you need to free your king if it is stuck on the eighth rank in front of the pawn? (Four.) How do you draw the game if you have the rook and your opponent has the rook and rook-pawn, and his rook is in front of the pawn? If the pawn is on the seventh rank, you want your rook behind the pawn, of course — that much is obvious. And you have to keep your king on the opposite side of the board from the pawn — something that is not obvious to a lot of players.
The thing I didn't know is what to do if the pawn is on the sixth rank. Silman shows that in that case you want your rook attacking the pawn from the side, not from behind, and he shows something called the Vancura position that I had never heard of.
This is all really cool stuff, but I do have one slight reservation. I've played loads of games, I believe, without playing a single game that went down to rook and rook-pawn versus rook. So it's unclear that knowing these endings will make a big practical difference to me (or to you). Also, the problem with learning rules like “you need four files between your king and the enemy king” is that in a game, you usually don't have control over this. The main thing to know is that you want to cut off the enemy king as far away from the passed pawn as possible. If you can get four ranks, then great. If you can only get three, then knowing that you need four is not going to be a big help to you.
Navier Stokes and smoothness
Basically, a function is differentiable iff it is continuous. Now if there exists a $k$-th derivative for a function, it means that the function is continuous at least $k-1$ times. They are using this to group functions into differentiability classes. A function of class $C^k$ has derivatives $f',f^{\prime\prime},\dots,f^{(k)}.$ Now, a smooth function is in the differentiability class $C^{\infty}$ which means that you can differentiate it as many times as you want. Which means it is always continuous.
Now that we know this, we should first try and understand that operator in Terrence Tao's paper $$\partial_t u=\Delta u+B(u,u)$$ and he says this $B$ is equivalent to the energy equation. As far as I understand, Tao doesn't assume the equation to be smooth, instead of $B(u,u)$ he assumed an averaged $\tilde{B}(u,u).$ And then he constructs a smooth solution and demonstrates finite-time blowup.
References: Navier-Stokes existence and smoothness on Wikipedia and Tao's paper: Finite time blowup for an averaged three-dimensional Navier-Stokes equation
Engine efficiencies and my attempts to relearn thermodynamics
Heat engines convert heat to energy, specifically mechanical energy, which can be used to move stuff around $(W=F\cdot\Delta x)$. It does this by using a substance to move things, while cooling the substance. A heat source initially heats the substance, the substance moves whatever it needs to move while losing heat. This substance is anything that has a heat capacity. Some heat is lost to the surroundings, and there are practical issues of friction and drag.
Note that heat pumps (refrigerators) do the exact opposite by performing work on a substance. They usually compress (do work) on a refrigerant (the working substance) and draw out heat. This is a diversion I do not wish to explore.
Carnot engines operate on the carnot cycle. The Carnot cycle has in order 1) isothermal expansion, (where it expands in volume by pulling in heat) 2) adiabatic expansion, (where it loses temperature because all the heat has to be dispersed among the expanding molecules) 3) isothermal compression (where it compresses by expelling heat) and 4) adiabatic compression where it gains temperature.
Lets see what happens if $Q_C=0$ (which means our engine doesn't lose any waste heat). Then we'll have negative entropy because $\Delta S=\displaystyle\frac{-Q_H}{T_H}<0$ which violates the second law of thermodynamics. Which means we have to consider waste heat. So the maximum work we can get is $\Delta W=Q_H-Q_C$. Now, considering both sources: $\Delta S=\displaystyle\frac{-Q_H}{T_H}+\displaystyle\frac{Q_C}{T_C}$ and we know that $\Delta S>0$. For the entropy to be positive, the minimum value of $Q_C$ will be $\displaystyle\frac{Q_H}{T_H}T_C$ and this means that $$\Delta W=Q_H\left(1-\displaystyle\frac{T_C}{T_H}\right)$$.
This means we can't have 100% efficiency because we can't exhaust the heat sink to 0 K, and we will definitely lose waste heat to the universe due to the second law of thermodynamics. Also, this is a purely theoretical concept, taking a Carnot cycle through its steps without losing any additional energy will require us to do it infinitely slowly, and it is impractical to build an engine that takes forever to do its job. Another way to think of the whole entropy argument before is to look at the S-T diagram and realize that for the cycle to complete we need to gain back all the entropy we lost.
Bonus: TIL Diesel is apparently the name of a dude called Rudolf Diesel who invented the Diesel Engine that runs on Diesel.
Automatic Content Selection
Content on the internet should be permanent, available, and addressable until the original author of the content chooses to remove it. Automatic Content Selection by Stephen Wolfram
- Even if you don't explicitly know something (say about someone), it can almost always be statistically deduced if there's enough other related data available
- Even given every detail of a program, it can be arbitrarily hard to predict what it will or won't do
- For a well-optimized computation, there's not likely to be a human-understandable narrative about how it works inside
- There's no finite set of principles that can completely define any
reasonable, practical system of ethics
Scaling Carbon Capture
- As solar power gets cheaper and oil becomes more scarce, at some point this decade it will be cheaper to electrically extract carbon from the air than to drill mile-deep holes in the crust on the other side of the world.
- We ideally want a Haber's process for carbon dioxide
- CO$_2$ is usually a by-product, and it can't be used for fuel anymore than any other fully oxidized stable chemical can.
- We need to put back the energy that was released when it was produced. And then some more to remove the oxygen atoms.
- Sabatier reaction:
catalytically react CO$_2$ with
hydrogen, producing methane (CH$_4$) and water vapour.
- Doing this requires a very large supply of pure hydrogen, ideally generated electrolytically, which requires an enormous supply of electricity.
- $\Delta H=-165.0$ kJ mol$^{-1}$
- This happens at very high temperatures, so $T\Delta S$ is very high and $\Delta G$ will be positive
- Overall efficiency is at 15% to 35% at best.
- Three big challenges
- CO$_2$ concentration
- CO$_2$ reduction
- Electricity Cost
MSTD sets
I was reading this interesting paper by Hegarty discussing Some explicit constructions of sets
with more sums than differences and I found that the smallest set you could
construct was $\{0,2,3,4,7,11,12,14\}$ and it is not possible to construct smaller
sets (of course, you could create other sets from this one using affine
transformations).
For example, take the set $\{2,5,6,8,11\},$ the sumset will be $\{4, 7, 8, 10, 11,
12, 13, 14, 16, 17, 19, 22\},$ and the difference set will be $\{-9, -6, -5, -4, -3,
-2, -1, 0, 1, 2, 3, 4, 5, 6, 9\}.$ The sumset has 12 elements and the difference set
has 15 elements, so this set is not an MSTD set.
For a set with $n$ numbers, the sumset will have an upper bound of
$\displaystyle\frac{n(n+1)}{2}$ and the difference set will have an upper bound of
$n(n-1)+1.$ We
can understand this by drawing a matrix and seeing that for the sumset, we'll have a
symmetric matrix and for the difference set, we'll have a skew-symmetric matrix. We
just need to ignore the zeroes in the diagonal for the difference set. Because we
lose half the numbers from the sum-set, we need to construct MSTD sets by having as
many duplicate values in the difference matrix as possible. An example where both
are equal is the set $\{k,k+1,k+2,\dots,k+l\}$ which has the sumset
$\{2k,2k+1,2k+2,\dots,2k+2l\}$ and the difference set $\{-l,-l+1,-l+2,\dots,l\}.$
Both have a cardinality of $2l+1.$ Another example of a set where both are equal is
the set $\{a, b\}$ where the sumset will have three elements, and the difference set
will have three elements. The sumset will be $\{a+b, 2a, 2b\}$ and the difference
set will be $\{a-b, b-a, 0\}.$
def sumdiffset(data):
sumset = set()
diffset = set()
for num in data:
for other_num in data:
sumset.add(num + other_num)
diffset.add(num - other_num)
return {'sumset': sumset, 'diffset': diffset, 'set_counts': {'sumset': len(sumset), 'diffset': len(diffset)}}
data = [0, 2, 3, 4, 7, 11, 12, 14] # change this
result = sumdiffset(data)
print(f"Sumset: {result['sumset']}")
print(f"Diffset: {result['diffset']}")
print(f"Set Counts: {result['set_counts']}")How do we lose body mass?
-
People who wish to lose weight while maintaining their fat-free mass are, biochemically speaking, attempting to metabolise the triglycerides stored in their adipocytes. The three most common fatty acids stored in human adipose tissues are oleate (C18H34O2), palmitate (C16H32O2), and linoleate (C18H32O2), which all esterify to form C55H104O6.
-
The complete oxidation of a single triglyceride molecule involves many enzymes and biochemical steps, but the entire process can be summarised as: C55H104O6+78O2→55CO2+52H2O+Energy.
-
Stoichiometry shows that complete oxidation of 10 kg of human fat requires 29 kg of inhaled oxygen producing 28 kg of CO2 and 11 kg of H2O. This tells us the metabolic fate of fat but remains silent about the proportions of the mass stored in those 10 kg of fat that depart as carbon dioxide or water during weight loss.
-
To calculate these values, every atom's pathway out of the body was traced using labelled heavy oxygen (O18).
-
A triglyceride's six oxygen atoms will therefore be shared by CO2 and H2O in the same 2:1 ratio in which oxygen exists in each substance. In other words, four will be exhaled and two will form water. Note that the Da stands for dalton, a unit of mass.
-
The proportion of oxygen that becomes CO2 is (661 Da (C55)+64 Da (O4))/(861 Da (C55H104O6))$\times$100=84%
-
The proportion of mass that becomes water is (105 Da (H104)+32 Da (O2))/(861 Da (C55H104O6))$\times$100=16%
-
Calculations show that the lungs are the primary excretory organ for fat. Losing weight requires unlocking the carbon stored in fat cells, thus reinforcing that often heard refrain of “eat less, move more.” The authors recommend these concepts be included in secondary school science curriculums and university biochemistry courses to correct widespread misconceptions about weight loss.
Links from the Winter of '23
The Art of
Distraction: Creativity, memory and our relationship with time
Youngest World Champion, Aditi
Swami
Following the money behind
Premier League betting sponsors
Algorithms for
Toddlers
Luis Caffarelli
has won the 2023 Abel Prize
50th
anniversary of the OEIS a New York Times profile by Siobhan Roberts
Epicycle clock in vector
graphics and animated time offsets by Sophie Houlden
Bad Science and Room
Temperature Superconductors: what a put-down!! how not to do science.
A Mechanical Binary
Adder!
Embedded
Möbius strips made out of paper can only be constructed with an aspect ratio
greater than $\sqrt3$
The 8000th Busy Beaver number eludes ZF
set theory
Joseph
Brodsky's 1987 Nobel Lecture
CAR-T therapy forces
autoimmune diseases into remission
A bell that rings two notes at
once
Wavy
brick walls use fewer bricks than straight walls
F. Scott Fitzgerald in Tender is the Night
“See that little stream — we could walk to it in two minutes. It took the British a month to walk to it — a whole empire walking very slowly, dying in front and pushing forward behind. And another empire walked very slowly backward a few inches a day, leaving the dead like a million bloody rugs. No Europeans will ever do that again in this generation.”
“Why, they've only just quit over in Turkey,” said Abe. “And in Morocco —”
“That's different. This western-front business couldn't be done again, not for a long time. The young men think they could do it but they couldn't. They could fight the first Marne again but not this. This took religion and years of plenty and tremendous sureties and the exact relation that existed between the classes. The Russians and Italians weren't any good on this front. You had to have a whole-souled sentimental equipment going back further than you could remember. You had to remember Christmas, and postcards of the Crown Prince and his fiancée, and little cafés in Valence and beer gardens in Unter den Linden and weddings at the mairie, and going to the Derby, and your grandfather's whiskers.”
“General Grant invented this kind of battle at Petersburg in sixty- five.”
“No, he didn't — he just invented mass butchery. This kind of battle was invented by Lewis Carroll and Jules Verne and whoever wrote Undine, and country deacons bowling and marraines in Marseilles and girls seduced in the back lanes of Wurtemburg and Westphalia. Why, this was a love battle — there was a century of middle-class love spent here. This was the last love battle.”
[wip] Some ideas on improving semantic segmentation with SAM
Use some pre-existing models like DeepLab and OneFormer as baseline semantic segmentation models. Get bounding box prompts by using semantic segmentation masks from the baseline models. Use the prompts to get SAM masks, these masks are not labelled well. Now use IoU score between both masks to label the SAM masks, and we end up with labels from the baseline models and masks from SAM. Test this hypothesis on around 100 images per dataset
Division Algorithm Theorem
Theorem: For all $a,b\in\mathbb{Z},$ with $b>0,$ there exists unique $q,r\in\mathbb{Z}$ such that $a=bq+r$ with $0\le r<b$
Proof: Basically, $q$ is the quotient and $r$ is the remainder. Consider the set $S={a-bx|x\in\mathbb{Z},a-bx\ge0}$. For this set, we will first choose $a, b$ and vary $x$ to get elements of the set.
In general, to find the smallest element $r$ in $S$, we have to argue that the set $S$ is not empty.
Claim: $S\neq\phi$
Case 1: $a\ge0$, we set $x=0$, so that we get $a-b(0)=a\in S$. The set is not
empty
Case 2: $a<0$, we will set $x=a$, so that $a-ba=a(1-b)\ge0$. The set is not empty
Let $r$ be the minimum value of $S$ and $q$ be the corresponding quotient for this value of $r$.
We have $r=a-bq$. Towards the contradiction, assume that $r\ge b$
$r=a-bq\ge b$
$r-b=a-b(q+1)\ge b-b\ge0$
This means that $r-b\in S$ because $r-b=a-b(q')$ where $q'=q+1$. But on the other hand, $r-b<r$ which contradicts our assumption that $r$ is the minimum value of $S$. This implies that $0\le r<b$
Now, all that is left is to prove the uniqueness of $q$ and $r$. Suppose that $q,q',r,r'$ are such that $a=bq+r=bq'+r'$. Assume $r'\ge r$, this assumption can work either ways. We have $b(q-q')=r'-r$. The LHS is a multiple of $b$ and the RHS follows the inequality $o\le r'-r<b$. The only way both can be true is if LHS $=$ RHS $=0$. This implies that $q=q'$ and $r=r'$. $\blacksquare$
1989 (Taylor's Version) is out!
✨🫶 My name is Taylor and I was born in 1989 🫶✨https://t.co/klomIqGx38 pic.twitter.com/sWofWRjpvN
— Taylor Swift (@taylorswift13) October 27, 2023
How do you know what you care about?
This was an answer I wrote to a friend that asked me how we're supposed to know what
we care about before we choose to work on something? These were my
thoughts...
The first heuristic that comes to mind is Feynman's. What do
you find yourself treating like play? I always think
that when you feel like you have to separate "work" from
"free-time", you're not doing
something that you care about. Because when you're working on stuff you care
about, every minute is literally
free time.
Heuristic 1: What we like to play with is what we care about most.
There are certain things you can't do without thinking a few standard deviations differently to your peers. For example, academic or industrial research, founding startups, creating art et cetera. This implies that the barrier to entry is sufficiently high for these fields of work. Crossing this barrier to entry implies that you really care about what you're doing. Heuristic 2: An inclination to do things with an unusually high barrier-to-entry.
Specific curiosity. Everyone has general curiosity about lots of things, but the curiosity is usually superficial and limited to one or two layers of understanding. When you care about something, you will display specific curiousity. For example, when I wanted to get better at shooting the football, I asked lots of people about lots of parameters, from their weight training regimen to the kind of shinpads they wore in the hope that I get better at it. Heuristic 3: You display an intense level of curiousity when you care about something.
Corollary to heuristic 3: The feeling of being a newbie never leaves you when you care about something. You are always aware that there's more to learn. Heuristic 3.1: You care about things you're most insecure about.
Book 22, The Odyssey
You thought I would never return from Troy;The entire book leads up to these lines and when they finally arrive, they are so delicious!!
and so - you dogs - you sacked my house, you forced
my women servants to your will and wooed
my wife in secret while I was alive
You had no fear of the undying gods,
whose home is spacious Olympus, and no fear
of men's revenge, your fate in days to come
Now all of you are trapped in death's tight embrace.
Katalin Kariko and UPenn
The University of Pennsylvania is acting proud of Katalin Karikó now that she's won a Nobel. But they kicked her out of her research assistant professor job when she insisted on doing the work that won her that prize:
"She recalls spending one Christmas and New Year’s Eve conducting experiments and writing grant applications. But many other scientists were turning away from the field, and her bosses at UPenn felt mRNA had shown itself to be impractical and she was wasting her time. They issued an ultimatum: if she wanted to continue working with mRNA she would lose her prestigious faculty position, and face a substantial pay cut.
”It was particularly horrible as that same week, I had just been diagnosed with cancer,” said Karikó. “I was facing two operations, and my husband, who had gone back to Hungary to pick up his green card, had got stranded there because of some visa issue, meaning he couldn’t come back for six months. I was really struggling, and then they told me this."
"While undergoing surgery, Karikó assessed her options. She decided to stay, accept the humiliation of being demoted, and continue to doggedly pursue the problem. This led to a chance meeting which would both change the course of her career, and that of science."
Elsewhere she recalled:
“I thought of going somewhere else, or doing something else. I also thought maybe I’m not good enough, not smart enough."
She's now an adjunct in UPenn's neurosurgery department. Will they fast-track her for tenure now that she has a Nobel, or just live with the shame?
Both quotes here come from interesting stories. The first is from here:
https://www.wired.com/story/mrna-coronavirus-vaccine-pfizer-biontech/
The second is from here:
https://billypenn.com/2020/12/29/university-pennsylvania-covid-vaccine-mrna-kariko-demoted-biontech-pfizer/
- John Carlos Baez (@johncarlosbaez) October 03, 2023
Links from the Monsoon of '23
Machiavelli: A Very Short
Introduction by Quentin Skinner
Ferrari vs. Mercedes along Eau
Rouge, 2017 to 2019
Manchester United vs.
Barcelona, UEFA Champions League Final,
Wembley 2011. Tiki-taka masterclass by Pep Guardiola.
Mechanical Watch by Bartosz
Ciechanowski
The Physics of Racing by
Andre Marziali
What
Does Any of This Have
To Do with Physics? by Bob Henderson
How
to Be a Mathematician (or
Theoretical Computer Scientist) by Ryan O'Donnell
Math Has a Fatal Flaw by
Veritasium
The Silver War, 2014 and
2016
The
Real Problem at Yale
Is Not Free Speech by Natasha Dashan
Joe Gebbia with Tim
Ferriss
Last of the Czars: Part 1: Nicky and
Alix, Part 2: The Shadow of
Rasputin, Part 3: The Death of the
Dynasty
36 all-out to Mission
Melbourne, The Great Sidney
Robbery and The
Gabbatoir Breach. The greatest Test series win,
relived by Ravichandran Ashwin and R. Sridhar.
Tribute to Christopher
Hitchens by The 2012 Global Atheist
Convention
the future linus
torvalds
Max-bounds on information travel speeds
You know how the light we see from stars was emitted ages ago and we're only seeing
the past because of the distance.
How do you find out whether a star exists at this moment in time? Because
information can't travel faster than the speed
of light, we are fundamentally constrained.
Let's talk about the sun because it's closer to us, and more intuitive to think
about. The sun is 499 light-seconds away
from us (what an annoying number) and we want to find out if the sun actually exists
now or if something happened to it
in the 499 seconds since it last emitted light. One thing we could do is to send
someone up to the sun and report back
with real time updates (ignore the fact that the sun would burn up this person, or
if you can't ignore it, imagine a
heat-proof sensor or something). But this fails because the fastest way the person
would send us the information would
still be slower than the speed of light.
After more failed thought-experiments, I thought of one that I can't seem to refute.
Tie someone to a rope that is
exactly the distance between the sun and the earth and fly them out to the sun. When
they reach the sun, the rope will
be taut. Now, when the sun disappears, all this person has to do is to pull on the
rope which will instantly alert us,
499 seconds before things actually go dark suddenly. Obviously, I'm ignoring
something, because nothing actually can
travel faster than the speed of light.
What am I missing? The only thing I can think of is that pulling on the rope doesn't
transmit forces instantaneously as
I hope, but why would it not be instantaneous? It's a rope, why will the length or
anything else matter? I'm being
incredibly myopic about something, but can't figure out what.
Update: It
takes time for an impulse to travel along a physical object. This can be seen with
high-speed differential cameras. I feel like I've won the Nobel Prize for Stupid.
rant: linkedin
In the everlasting spirit of keeping things petty here, let's talk about LinkedIn. How many times have I had people I've barely known ask if I could "connect" (what a repulsive word) on that abomination of a site and I felt every shred of respect leave my body. LinkedIn needs to be mocked mercilessly for its assumption that our worth as humans is solely tied to the work we've done. It needs to be ridiculed for how it functions as a platform for people to continually debase themselves in public. It is a humiliation ritual, that people willingly take part in. Actually, no, scratch that, I don't think there has been a single person that woke up and went, "let me create a linkedin account today" So what is it? What is it that makes the most normal person you know get "beyond excited" to tell the world about their leadership skills or be "thrilled" (seriously, do people even know what this word means?) to explain how they "create value". The most honest answer given to me when I asked a friend was that having an account makes it easier to stalk other people on LinkedIn. At least that's a noble cause.
Few things are more icky than people using social media to write motivational quotes and reposting company posts in order to show how much they love their new job. It just doesn't matter, so close your account and find platforms where people get to know you as something more than the number of connections you have (if that's what is important on LinkedIn). Don't become a career.
2024 Breakthrough Prize
Simon Brendle, Columbia University. For transformative contributions to differential geometry, including sharp geometric inequalities, many results on Ricci flow and mean curvature flow and the Lawson conjecture on minimal tori in the 3-sphere.
A Matter of Concern...
Scientists publish studies on how too much sitting will kill you, and how standing desks aren’t much better … but how about a study of people who plant their feet against the nearest surface and tip back and forth on their chair, the way their teachers always told them not to?
- Greg Egan (@gregeganSF) September 08, 2023
rant: short form video content
Notice how short-form video clips taken from longer videos/interviews used to be
clips, then they became clips with
subtitles, then clips with coloured/highlighted subtitles, and now they have
background music or special effects added.
This will only get worse.
Short form content is so repulsive to me, it is an incremental commoditization of
our attention. A relentless attack on
our senses, until the baseline is far removed from the norm. I'm going to write an
essay about this if I can sufficiently marshall my attention.
The Making of the Atomic Bomb
Finished reading The Making of the Atomic Bomb and it's a solid 5/5. Richard Rhodes
gets the balance -between writing
science, and the human stories- perfect. All the brilliant scientists I'd grown up
reading about come together and their
science fits in like a perfect mosaic to split the atom.
The chapter on the bombing and effects on the Japanese cities is traumatic to read,
a reminder about man's inhumanity
towards man. Reminded me of Voltaire's, "It is forbidden to kill; therefore all
murderers are punished unless they kill
in large numbers and to the sound of trumpets."
When you read Feynman's accounts, you have this impression that life at Los Alamos
revolved around him. So it was
surprising to me that he's only mentioned thrice in the entire book, and only one of
those times, he's described as
doing anything (setting up the radio before the Trinity test)
In chronological order, the architects of our understanding of the atom. Democritus,
Aristotle -> Newton, Dalton,
Avogadro -> Thomson -> Rutherford (nucleus) -> Plank, Einstein, Bohr -> Rutherford
(proton), Chadwick -> de Broglie,
Schrödinger, Heisenberg.
And for the splitting the atom. Becquerel, Curie, Rutherford -> Fermi -> Hahn,
Meitner, Frisch -> Szilárd -> Bohr,
Anderson -> Teller, Wigner, Einstein-Szilárd -> Roosevelt, Oppenheimer, Groves,
Manhattan Project.
The Shoulders of Giants.
Für Therese
Wow! I never knew that Beethoven's "Für Elise" was probably written for a woman named Therese - and the letters these two names have in common:
E S E
may have inspired the alternating sequence of notes that starts this piece:
E E♭ E
Yes: in German E flat is called "Es" and pronounced like the letter S.
I also didn't know that Beethoven never published "Für Elise" in his life: he gave the score to Therese Malfatti, and it was transcribed and published by someone else after his death, and hers. Beethoven almost published a second more complicated version of the tune in 1822... but at the last minute decided not to.
I learned all this stuff from this video, which also includes a performance of the second version of "Für Elise". If you like the famous version, which is rather simple, this one may seem ludicrously fancy. But it's interesting.
In 1810, Beethoven wrote a letter which ended like this:
"Now fare you well, respected Therese. I wish you all the good and beautiful things of this life. Bear me in memory—no one can wish you a brighter, happier life than I—even should it be that you care not at all for your devoted servant and friend, Beethoven."
https://www.youtube.com/watch?v=jblFQ1whX5s
- John Carlos Baez (@johncarlosbaez) August 17, 2023
Intermediate activations in Llama 2.7B
By layer 24, the model is quite certain about the correct answer, and the remaining computations are mostly redundant, mainly re-weighting alternative less obvious completion paths such as 'The capital of Germany is {a, the, one, home, located...}'. Interestingly, the model becomes less certain about 'Berlin' from layers 24-31 as it figures out more alternative options. The attention output of layer 24 of the llama 2 transformer consistently represents relevant information related to countries, even when neither the prompt nor the higher probability completions are related to countries
the inexorable march of time...
can someone tell me where the phrase, "the inexorable march of time" originated? I
absolutely love it, but can't seem to find who came up with it. G. K. Chesterton has
"relentless march of time" and Walt Whitman has "long march of time".
at my wits' end...
Update: I asked this on Mathstodon, and Prof. Michael Kinyon from the University of
Denver found an 1876 reference
by a R. W.
Shufeldt of the U. S. Navy.
Notes on Nausea
Prodromal phase of emesis: Salivation increases, sometimes a lot, pupils dilate, heart races, blood vessels constrict, cold sweat. Attention and focus go down, and fatigue starts to set in. Stomach has electrical activity, and relaxes to prevent emptying. Oesophagus contracts to pull the stomach into the chest through the diaphragm (like a funnel). Retrograde giant contraction: small intestine pushes contents back to the stomach. In the lower small intestine, contents are pushed to the colon
Vomiting act: Retching involves muscles of the abdomen, oesophagus and respiratory system, but nothing comes out. During expulsion, intense pressure is generated in the stomach after the diaphragm and the abdomen contract
Nausea: From the Greek word meaning ship. Intensely aversive, used
in behavioural change therapy (rather controversially). Aside: potatoes during
pregnancy is a bad idea, unless you want your child to have a neural disease.
Pregnancy nausea as a evolutionary defence mechanism, because the embryo is
sensitive to toxins in food. Women with moderate to severe emesis during pregnancy
have lesser miscarriages than women with mild to no emesis. But we don't know
why nausea happens with motion sickness.
Motion sickness happens when the
motion that we experience is not the motion that we are expecting. No convincing
explanation for why anxiety, or the sight of blood, or the sight of vomit causes
nausea. Injury from sever vomiting can occur: rupture of the oesophagus, lung
collapse, tearing of the spleen. Some doctors think pregnancy sickness is due to
unconscious rejection of pregnancy (originally touted by Freud). Vomiting and nausea
are not connected always, you can have one without the other, considering how common
this is, we still don't have a good antiemetic drug.
John Bardeen
To understand what LK99 was all about, I was looking at how superconductors work
(revising the basics) and I found out
that John Bardeen came up
with the Theory of Superconductivity. The John Bardeen!
The very same man that invented the Transistor with William Shockley!! He's the only
person to win the Physics Nobel twice.
Wow, superconductivity AND the transistor. Where does he stand in the most
influential person of all time rankings?
Symmetry and Newton's Laws
A little classical mechanics problem you can solve without doing any calculation:
Consider the hyper-simplified problem of a bell-shaped hill, and a point rock that can slide without friction up and down the hill. If you start with the rock at the bottom, and give it exactly the kinetic energy needed to arrive to the top and stop there without sliding on the other side, how long will it take to arrive there?
- j_bertolotti (@j_bertolotti) August 03, 2023
Allen Mandelbaum's translation of The Odyssey
Muse, tell me of the man of many wiles,
the man who wandered many paths of exile
after he sacked Troy's sacred citadel.
He saw the cities—mapped the minds—of many;
and on the sea, his spirit suffered every
adversity—to keep his life intact,
to bring his comrades back. In that last task,
he will was firm and fast, and yet he failed:
he could not save his comrades. Fools, they foiled
themselves: they ate the oxen of the Sun,
the herd of Hélios Hypérion;
the lord of light requited their transgression—
he took away the day of their return.
Muse, tell us of these matters. Daughter of Zeus,
my starting point is any point you choose.
All other Greeks who had been spared the steep
descent to death had reached their homes—released
from war and waves. One man alone was left,
still longing for his home, his wife, his rest.
For the commanding nymph, the brightest goddess,
Calypso, held him in her hollow grottoes:
she wanted him as husband. Even when
the wheel of years drew near his destined time—
the time the gods designed for his return
to Ithaca—he still could not depend
upon fair fortune or unfailing friends.
While other gods took pity on him, one—
Poseidon—still pursued: he preyed upon
divine Odysseus until the end,
until the exile found his own dear land.
Conway's Game of Life is Omniperiodic!
The first period-41 oscillator in Conway's Game of Life was just found by Nico Brown: https://conwaylife.com/forums/viewtopic.php?p=163972#p163972
With that, oscillators of every period are known: Conway's Game of Life is omniperiodic! This has been one of the major open problems in the study of Conway's Game of Life even since its invention over fifty years ago.
This comes just 7 days after the second-to-last period, period 19, was discovered.
- Izzy Grosof (@isaacg) July 22, 2023
IMO 1988 Day 2, Question 6
Let $a, b\in\mathbb{Z}^{+}:ab+1 | a^2+b^2.$ Show that
$\displaystyle\frac{a^2+b^2}{ab+1}=r^2$
where $r\in\mathbb{Z}$.
I figured out that the solutions were $a=n,b=n^3,$
and then concluded falsely that that was it. Turns out there are infinitely more and
the only reason that $(n,n^3)$ shows up as the first solution is a coincidence of
the complete proof.
Zvezdelina Stankova explains on Numberphile one solution
that uses induction with infectious enthusiasm. This problem consumed me, and its
insides were wondrous.
John Conway's Doomsday Algorithm
Pi day (March 14) and the last day of February is Doomsday.
Apart from February, the $n$th day of the $n$th month is
Doomsday for all even months.
Switch dates for months 9, 5 and 7, 11. We already know March. January has Doomsday
on the 3rd on non-leap years and on the 4th on a leap year.
Doomsday advances by one day each year because 365 divided by 7 leaves 1 remainder.
Doomsday advances two days each leap
year.
Speak Now (Taylor's Version) is out!
It's here. It's yours, it's mine, it's ours. It's an album I wrote alone about the whims, fantasies, heartaches, dramas and tragedies I lived out as a young woman between 18 and 20. I remember making tracklist after tracklist, obsessing over the right way to tell the story. I had… pic.twitter.com/I2cLH76EIH
— Taylor Swift (@taylorswift13) July 7, 2023
John B. Goodenough
John Goodenough and his work on
Li-ion batteries and computer random-access-memory.
Immortal.
"a product that today touches nearly everyone's life"
Links from the Summer of '23
2020's biggest breakthroughs in Math and Computer
Science, Physics, and Biology
Taylor Swift:
folklore, evermore and
Songwriting
Who goes Nazi? by
Dorothy Thompson (1941, Harpers)
Dan Carlin on the Lex Fridman
podcast.
Essay:
How do you describe TikTok?,
"The best bodily position in which to watch TikTok is supine, muscles slack,
phone above your face like it's an
endless tunnel into the air."
Trench verses by Belash Yuri
Semyonovich
The Insect
Brain
A
Mathematician's Lament by Paul
Lockhart
Against Dog
Ownership
Documentary on Amal Kumar
Raychaudhuri
The Offensive Lineman -
with John Urschel on the
Numberphile podcast.
A survey of some famous
problems in
Mathematics over the years, compiled by Alvaro Rozano-Robledo.
A perfect Suzuka qualifying
lap by Sebastian Vettel in the
AMR22.
some
cool problems to think about... in matrix completion from LessWrong
Gilbert Strang's Final
Lecture, streamed live by MITOCW. Brilliant as usual.
Harvard Careerism
(From The
Crimson)“Students that don't come from familial wealth may be “disinclined
to smell the
flowers along the way and to take some
chances,” said former Dean of Freshmen Thomas A. Dingman '67.”
“The clearest example of Harvard's careerist turn is its extracurriculars… student
organizations… increasingly function
as pre-professional outlets.”
Key Changes
Apparently, there are lesser key changes in the billboard top 100 nowadays due to 1)
the
rise of hiphop (which doesn't
really care about keys as much as it cares about rhythm and lyrics) and 2) digital
recording (which doesn't constrain
artists to use instruments like guitar and keyboard only). One exception is Sicko
Mode
by Travis Scott and Drake. Also
discussed was Michael Jackson's Man in The Mirror and the key change near the start
of
the second chorus.
From Chris Dalla Riva on Tedium.
Nicholas Mahut on Rafael Nadal's dominance at Roland Garros
When you play Roland Garros 14 times you tell yourself you had a good career. When you win 14 matches there, that's not too bad at all. When you get to the second week 14 times you are one of the great players. And when you win the title 14 times, there is no way to comprehend that. There are no words.
Informed Consent
Are patients even capable of providing "informed consent" before a medical procedure? The keywords being informed, and consent. Considering how less the layman knows about the human body compared to a trained medical doctor. What does it mean if they can't? Both legally and ethically.
Ethics of AI cloning
Should it be illegal to make software that allows the AI cloning or deep faking of the voice, image, or video likeness of real people without their consent?
— Katherine Brodsky (@mysteriouskat) May 18, 2023
Verstappen Rising
There's no stopping Max this season, he's just breezing past everyone. I guess F1 has converged to a point where aero is so overpowered that there wont be any competition if you get it right. This isn't even recent phenomena. It started with Sebastian and then Lewis and now Max. One car, and usually one driver (that's in tune with that car) dominating the field.
Get Some Free Speech While You Can
Freedom of expression isn't the tool of the powerful; it's the tool of the
powerless. From Caitlin Flanagan's Atlantic article.
Malcolm Gladwell pointed out the absurdity of her position by tweeting, “I signed
the Harpers letter because there were
lots of people who also signed the Harpers letter whose views I disagreed with. I
thought that was the point of the
Harpers letter.”
Whenever a society collapses in on itself, free speech is the first thing to go.
That's how you know we're in the
process of closing up shop. Our legal protections remain in place—that's why so many
of us were able to smack the Trump
piñata to such effect—but the culture of free speech is eroding every day. Ask an
Oberlin student—fresh outta Shaker
Heights, coming in hot, with a heart as big as all outdoors and a 3 in AP Bio—to
tell you what speech is acceptable, and
she'll tell you that it's speech that doesn't hurt the feelings of anyone belonging
to a protected class.
Field Trip to Citi Neuro Hospital!
Today, we went to Citi Neuro Hospital with everyone taking the Medical Image Analysis course. There were ten of us and we booked an SUV to the place. The air was super hot outside the campus, and the first thing I noticed about Hyderabad was how undulating the terrain was. Apart from small areas of the city, most of it is super uneven. The place we went to, Banjara Hills, is literally that, hilly. Some parts of the roads were like roller-coasters, steep and winding.
Banjara Hills as a residential area is pretty posh, and I definitely might have seen some A-list celebrity homes while I passed them. We went to the hospital and I took out my mask (haha). Everyone else had to buy masks. I wish I looked more closely at the patients (the hospital was CROWDED) because this wasn't like a usual hospital, it was for neuro disorders, so I'm sure the patients would be remarkedly different. In general hospitals, people usually look pained, and in suffering. Anyways.
Dr. D. Ravi Varma who's part of the board (and he probably owns the hospital) came out to receive us. The staff and the patients gave us a wide berth when they saw who we were with. He took us first to the X-ray room. It was kinda fancy, he showed us how the walls were super thick so that radiation wouldn't leak outside, and then I saw that some walls had water seeping through them and I looked very suspiciously at that for a while. Because I was trying to think whether water would be affected by X-rays in any way. The X-ray machine was super sophisticated, it could move in two directions (say X-axis and Y-axis) and could rotate along one plane.
The room had provisions for a full body X-ray (where the patient has to lie down) and for a chest X-ray (where the patient has to stand, much like Roentgen's wife in the original X-ray experiments). I didn't really understand why the two are so different, even though he said something about magnification. The operator stands behind a lead frame? (the right word evades me at the moment) and the glass he sees through also has lead in it. There was a heavy (I tried it on, it is heavy) lead apron for pregnant women so that the foetus is not harmed.
Then we went to CT, the machine costs 1.2 crores, and they're planning to replace it with a machine that costs 3 crores. CT was pretty uneventful, except that I saw a warning on the CT machine that warned technicians to not put their fingers in the machine. I mean, some idiot must have done that for the machine to come with that warning. Also, for angiography, they use iodine (which is super dense) in order to mark out blood flow. But the body only wants limited quantities of iodine, and injecting that much iodine is super bad. So they tie up iodine in a molecule called iodohex? me forgot :(
Then we went underground, which really puzzled me, because the hospital was like 5 stories high or something. Guess why? These MRI machines are so heavy, so heavy, that the roof would fall on your head if you placed it on standard slabs. Being a civil engineer, you could see how interested I was in all this. 6 tonnes, is how heavy the machine is. And the machine was a thing of beauty. Physics and engineering in perfect tango. We had to remove all metallic objects and we went inside. There were pictures of the beach inside in all directions to help claustrophobic patients. I asked how many claustrophobic patients he gets, and he said, VERY SURPRISINGLY TO ME, that almost all the patients he gets are claustrophobic.
We spent so much time with the MRI machine, Ingenia 3.0 Tesla by Philips. It has some 3 million miles of wire about 2 micron thick running with some huge current to generate the magnetic field. The current is sustained by ensuring superconductivity of the material, liquid Helium is sustained at 4K (-269 C) by using a heavy Carnot engine, that pumps so loudly that the room vibrates constantly (thud, chain click, thud, chain click). The patient is given a pair of pneumatic headphones (which are super awesome, they convert digital sound to pneumatic sound, and the inside of the wires have loads of cilia, which propagate the sound) so that they're not affected by the magnet. I wish I asked if the patients could choose their own music.
I was fascinated by the warnings, and the backups, and the backups to the backups they had. One warning near the MRI machine showed a person being sucked into the machine because they got too close with their wheelchair, the drawing was hilarious. There were 3 separate buttons to turn off the magnet in case of such a situation. Apparently, turning of the magnet and turning it back on will cost, wait for it, drum roll, 2 crores. Self-explanatory no? THAT IS MORE THAN THE CT MACHINE.
Phew, once, they lost power supply to the machine because of some circuit breaker. An MCB, it didn't break all the way and got stuck in the middle, and because of that, they didn't get power from either the electricity board or their generator, and the machine turned off. Since then, they have installed a protocol where they have sensors that always tell them whether the board is giving them power or not. And if that protocol fails, the chief doctor (Ravi Varma in this case) has a direct line to the EB (electricity board) in charge with priority. All rather important stuff.
When I said underground, you should have thought of what I said earlier, that we were in Banjara Hills. The hospital has been built into a hill. Dug into rock, I wonder how stable the building really is. It has been 7 years, and I definitely did see some cracks in some corners. He then showed us how fast his computing machines were (they have state of the art GPUs to get instantaneous reconstructions in 3D, and in super high resolution), their data center, which gets filled by 1TB a year (that is unreal) because of all the high-quality images they store. That is that, I don't think I've forgotten anything else. One funny thing that happened was one student asked him how long the generator runs, and he replied rather drily, "as long as we pour diesel into it".
I found it cool, how well-versed he was with the physics, the engineering, the computation, the algorithms, and the logistics of his hospital. He's a doctor, and he's the first one I've seen that I would never guess was a doctor unless he told me.
Exponential improvement for the bound for diagonal Ramsey numbers
I was at an amazing seminar yesterday in Cambridge, where Julian Sahasrabudhe announced that he, Marcelo Campos, Simon Griffiths and Rob Morris have obtained an exponential improvement for the bound for diagonal Ramsey numbers.
The preprint came out on arXiv today. It is 57 pages but written in a relaxed expansive way (he tells me). I imagine there will now be a flurry of developments as people digest the argument, which is surprisingly elementary (e.g. it doesn't make use of quasirandomness). It was one of my favourite problems, but I would never have found this argument, so am very happy to see it solved. Huge congratulations to all involved.
— Timothy Gowers (@wtgowers) March 17, 2023
Links from the Spring of '23
Read Something Great
Save matplotlib figures as
TikZ/PGFplots for smooth integration into LaTeX
Queen and Rook Endgames: A
Study on LiChess
Forensics
of the Subclavian Vein
The
Confessions of Marcus Hutchins on Wired
Steering
into the Iceberg on Common Sense with Dan Carlin
$\LaTeX$ pipeline
On Unix, install texlive-full for compiling TeX, vi as
code editor, and zathura as document viewer. Running
latexmk -pdf -pvc file.tex will open a .pdf file everytime
file.tex is saved. EB Garamond is unmatched for typesetting
mathematics.
Thoughts on Amnesia
Patients with amnesia or agnosia usually answer questions by cues rather than memory, a sort of inference. For example, identifying the month by looking out the window and identifying platonic solids by feeling them. Is the loss in these pattern making abilities greater nowadays than before? What can we recognize nowadays by cues? shapes, yes, I imagine. Seasons, maybe not? More auditory cues than haptic cues?
The Human Placenta
Placental protein 13 creates a diversion, it goes to some other part of the uterus that the placenta isn't trying to invade and attracts the attention of the immune system. Human placental lactogen can cause pre-eclampsia, dangerously high blood pressure leading to organ failure. By the third trimester, 25% of the blood flow is going into the placenta.
Links from the Winter of '22
Tom Lehrer songs archive
Life begins at 40: the biological and
cultural roots of the midlife crisis | The
Royal Society
Best of Nerdwriter1: Harry
Potter & The Prisoner of Azkaban:
Why It's The Best - YouTube, Mr. Bean Is A Master Of
Physical Comedy - YouTube, The Death of Socrates:
How To
Read A Painting - YouTube
Theory
Thursday sessions held weekly at IIITH
Reversals in psychology
Some fun math puzzles
Figure out the rule that governs the following sequence and the next term in the
sequence: 6, 3, 3, 4, 4, 7, 3, 9, 8, 3,
3, 4, 4.
Imagine a rectangular table made with "4920 $\times$ 2352" boxes, how many boxes
does the line joining the opposite
corners pass through?
Midnights is out!!
Midnights is a collage of intensity, highs and lows and ebbs and flows. Life can be dark, starry, cloudy, terrifying, electrifying, hot, cold, romantic or lonely. Just like Midnights. Which is out now
— Taylor Swift (@taylorswift13) October 21, 2022
Solutions to IYMC 2022 Qualification
Problem A: What are the roots of the function $f(x)=(\log(3^{x})-2\log(3))\cdot(x^2-1)$ with $x\in\mathbb{R}?$ $$f(x)=(x-2)(\log(3))\cdot(x^{2}-1)$$$$x=-1,1,2$$ Problem B: Find the values of the following infinite sum $$1+\frac{3}{\pi}+\frac{3}{\pi^2}+\frac{3}{\pi^3}+\frac{3}{\pi^4}+\frac{3}{\pi^5}+\cdots$$ We can rewrite the sum as $$1+\frac{3}{\pi}\bigg(1+\frac{1}{\pi}+\frac{1}{\pi^2}+\frac{1}{\pi^3}+\frac{1}{\pi^4}+\cdots\bigg)$$ Using the formula for the sum of geometric progressions and noting that $\displaystyle\frac{1}{\pi}<1,$ we can write $$1+\frac{3}{\pi}\bigg(\frac{1}{1-1/\pi}\bigg)=\frac{\pi+2}{\pi-1}$$
Multilingual Speakers
When a multilingual person wants to speak, the languages they know can be active at the same time, even if only one gets used. These languages can interfere with each other, for example intruding into speech just when you don't expect them. And interference can manifest itself not just in vocabulary slip-ups, but even on the level of grammar or accent.
Separation of languages happens by a suppression of the non-relevant languages. A kind of attention mechanism. The problem is called language-control in multilinguals. Errors are a great way to understand this. Sometimes, it seems like they inhibit the dominant language so much that they actually are slower to speak in certain contexts. A reversal of language dominance, where you end up making a mistake in your primary language
Wow, Spanish and English errors have "pero" and "but" as something common. I personally have shifted to the understanding part of language with that particular word.
Through the use of eye-tracking technology, Gollan and her team found that these mistakes were made even when participants were looking directly at the target word. She explains that when mixing languages, multilinguals are navigating a sort of balancing act, inhibiting the stronger language to even things out - and sometimes, they go too far in the wrong direction
Sometimes bilinguals will produce the right word, but with the wrong accent, which is a really interesting dissociation that tells you language control is being applied at different levels of processing
As it turns out, the Italian migrants were more likely to reject correct Italian sentences as ungrammatical if these did not match correct English grammar. And the higher their English proficiency, the longer they had lived in Canada, and the less they used their Italian, the more likely they were to have found the correct Italian sentences ungrammatical
Act III Scene II, Julius Caesar
Friends, Romans, countrymen, lend me your ears;
I come to bury Caesar, not to praise him.
The evil that men do lives after them;
The good is oft interred with their bones;
So let it be with Caesar. The noble Brutus
Hath told you Caesar was ambitious:
If it were so, it was a grievous fault,
And grievously hath Caesar answer'd it.
Here, under leave of Brutus and the rest-
For Brutus is an honourable man;
So are they all, all honourable men-
Come I to speak in Caesar's funeral.
He was my friend, faithful and just to me:
But Brutus says he was ambitious;
And Brutus is an honourable man.
He hath brought many captives home to Rome
Whose ransoms did the general coffers fill:
Did this in Caesar seem ambitious?
When that the poor have cried, Caesar hath wept:
Ambition should be made of sterner stuff:
Yet Brutus says he was ambitious;
And Brutus is an honourable man.
You all did see that on the Lupercal
I thrice presented him a kingly crown,
Which he did thrice refuse: was this ambition?
Yet Brutus says he was ambitious;
And, sure, he is an honourable man.
I speak not to disprove what Brutus spoke,
But here I am to speak what I do know.
You all did love him once, not without cause:
What cause withholds you then, to mourn for him?
O judgment! thou art fled to brutish beasts,
And men have lost their reason. Bear with me;
My heart is in the coffin there with Caesar,
And I must pause till it come back to me.
Sebastian Vettel's Greatest Hits
38 laps on the soft, in Bahrain 2018
Ferrari screw ups in Australia 2016, Canada, Germany 2016, Germany 2018, Italy
2018,
China 2019, Russia 2019, Mexico
2019, Silverstone 2020 costing valuable points
Monza 2008 win
Brazil 2012 win
Most number of pole positions in a season (15) in 2011
Most number of wins in a season (13) in 2013
Most number of consecutive wins (9) in 2013
Youngest pole sitter in 2008
Youngest world champion in 2010
The Genius Of The Crowd, by Charles Bukowski
but there is genius in their hatred
there is enough genius in their hatred to kill you
to kill anybody
not wanting solitude
not understanding solitude
they will attempt to destroy anything
that differs from their own
not being able to create art
they will not understand art
they will consider their failure as creators
only as a failure of the world
not being able to love fully
they will believe your love incomplete
and then they will hate you
and their hatred will be perfect
like a shining diamond
like a knife
like a mountain
like a tiger
like hemlock
their finest art
Endgame puzzle
 White can win this endgame
with perfect play! It seems obvious now, that I need to move the white king all
over
to f6, while guarding the c pawn with the bishop. Then black's bishop will be
overloaded trying to defend the f pawn and also prevent the h pawn from moving
up.
But I drew this position while actually playing it by giving away my h pawn
after
which the position is equal, even though white has a pass pawn.
White can win this endgame
with perfect play! It seems obvious now, that I need to move the white king all
over
to f6, while guarding the c pawn with the bishop. Then black's bishop will be
overloaded trying to defend the f pawn and also prevent the h pawn from moving
up.
But I drew this position while actually playing it by giving away my h pawn
after
which the position is equal, even though white has a pass pawn.
Cristiano Ronaldo in The Player's Tribune
But when you're a kid, you don't care about money. You care about a certain feeling. And on that day, this feeling, it was very strong. I felt protected and loved.
When you lose, it's like you're starving. When you win, it's still like you're starving, but you ate a little crumb.
When I was 15, I turned to some of my teammates during training. I remember it so clearly. I said to them, “I'll be the best in the world one day.”
They were kind of laughing about it. I wasn't even on Sporting's first team yet, but I had that belief. I really meant it.
It is like a final reminder … a final motivation. It says, “El sueño del niño.” The dream of the child.
German names
Today I learnt that Michael Schumacher is pronounced as mika-el schoomahhhuh and Sebastian Vettel is pronounced zebaastyan fetl. Nice. The rabbit hole that led me there was Juergen Schmidhuber's blog on which he has a page on Schumi where he focuses on details about Schumi's money and fame rather than his race stats and achievements.
Maryam Mirzakhani anecdote
After completing an undergraduate degree in mathematics at Sharif University in
Tehran in 1999, Mirzakhani went to graduate school at Harvard University, where
she started attending McMullen's seminar. At first, she didn't understand much
of what he was talking about but was captivated by the beauty of the subject,
hyperbolic geometry. She started going to McMullen's office and peppering him
with questions, scribbling down notes in Farsi.
“She had a sort of daring imagination,” recalled McMullen, a 1998 Fields
medalist. “She would formulate in her mind an imaginary picture of what must be
going on, then come to my office and describe it. At the end, she would turn to
me and say, 'Is it right?'' I was always very flattered that she thought I would
know.”
2022 Fields Medals!!
James Maynard for distances between primes, existence of infinite decimal
representations of primes with no digit 7 (or any other digit).
Maryna Viazowska for sphere packing
in eight dimensions.
June Huh for proof that absolute values of coefficients in chromatic polynomials
are unimodal and log-concave. (chromatic polynomial for a complete graph is
$x(x-1)(x-2)\cdots(x-n+1),$ for a graph with no edges is simply $x^n,$ and for a
tree on $n$ vertices is $x(x-1)^{n-1}$)
Links from the Summer of '22
Some
cool math puzzles from Quanta Magazine
People
dislike their political opponents for views that most don't actually hold.
[N=4993] on Twitter
The Math Doctors
Wittgenstein
at War from The New Statesman
Rapoport's
Rules for debate from Daniel Dennett's "Intuition Pumps and Other Tools
for Thinking"
Botticelli's The Birth of Venus was inspired by Phyrne of
Thespiae
Your Local
Epidemiologist by Dr. Katelyn Jetelina. Paxlovid (88% reduction, 89%
efficacy) and Molnupiravir (30% reduction)
Complex-Valued Autoencoders for
Object
Discovery
published in Transactions on Machine Learning Research (TMLR)
The Art of the
Command
Line
Nicer ceil function in C++
The inbuilt ceil function was being very unwieldy. I prefer defining
my
own inline function now.
int ceil(int numerator, int denominator)
{
return (numerator + denominator - 1) / denominator;
// in case of suspected overflow
return numerator % denominator == 0 ? numerator / denominator : numerator / denominator + 1
}The Hellespont
The Hellespont is the ancient name for the Dardenelles Straits, they separated Europe from Asia. Alexander crossed the Hellespont from Greece and threw his spear into Persian soil, thereby declaring war on the Achaemenid Persian Empire. Personally, I think this act was what defined Alexander. Alexander could have stayed home in Greece, raised a family, been a great king, and died a celebrated man. But this was not him. By crossing the Hellespont and daring to invade Persia, he transcended his mortality. It is a reminder to me that I have a Hellespont to cross.
Productivity Culture
The fatal flaw in the productivity culture: Its mandate is never, “you figured out how to do my tasks more efficiently, so you get to spend less time working.” It is always, “you figured out how to do your tasks more efficiency, so you must now do more tasks.”
Links from the Spring of '22
The
Best of Dan's Narration from r/dancarlin
Brief
Intro to
Game Theory on SSRN
Date
Formatting in Javascript on StackOverFlow
The
Fading Battlefields of World War I from The Atlantic
Where do Birds Go? from xkcd
The
Machine Learning Community has a toxicity problem from
r/MachineLearning
Colour
photographs from The Great War, these are nothing like I expected them
to
be. Granted, the soldiers were posing but these photos give off an idyllic
vibe. Very unsettling.
Elon Musk and Sal Khan
talk
about Entrepreneurship on YouTube
Career Panel with Yoshua
Bengio and Richard Sutton on YouTube
Eagle's Nest, Hitler's
HQ
and all of Mark Felton's videos
GitHub
Stars are overvalued
Typesetting
before $\LaTeX$
Save matplotlib figures as
TikZ/PGFplots for smooth integration into LaTeX.
Nietzsche's "Morality is Cowardice"
If you don't have the courage to commit a crime, it doesn't mean you're moral for not committing a crime. It just means you're afraid of doing it. We can see this in mob violence, people riot because they don't think they'll get caught.
Thoughts on Privacy
People assume that if they're not doing anything unlawful, there is no threat to their privacy. If there is unlawful activity, it is all the more reason for the activity to not be private. I read some version of this thought on an SSRN paper, I can't recall which.
Some Kimi Räikkönen stats
Most number of fastest laps in a season (10) and third on the all-time list of
fastest laps (46)
Most number of podiums after not starting from the front row (72)
Only driver to win for McLaren, Ferrari, and Lotus
Only driver to win races in the three different engine regulations eras (3.0
litre
V10, 2.4 litre V8 and 1.6 litre V6
turbo)
If, by Rudyard Kipling
If you can make one heap of all your winnings
And risk it on one turn of pitch-and-toss,
And lose, and start again at your beginnings
And never breathe a word about your loss;
Modular Arithmetic
(a + b) % m = (a % m + b % m) % m is fairly obvious to derive.
(a * b) % m = ((a % m) * (b % m)) % m can be derived by writing out
the
multiplication as repeated addition.
Fermat's
Little
Theorem states that if $p$ is a prime, then for any $a\in\mathbb{Z},$
$$a^p\equiv a\mod p.$$
(a / b) % m = (a % m * b^-1 % m) % m can be derived from Fermat's
Little
Theorem. The multiplicative inverse of $b$ modulo $m$ is $b^{-1}$ such that
$b\cdot b^{-1}\equiv 1\mod m.$
How Many Recursive Calls Does a Recursive Function Make?
The paper looks a the number of function calls made to itself by a function calculating the Fibonacci numbers recursively. There is a linear relationship between the Fibonacci numbers and the number of recursive calls. The number of function calls for a number $n$, $G(n)$ is related to the Fibonacci number $F(n)$ by the relation $G(n)=2F(n)-1.$ Achieved by adding a global counter to the recursive function and incrementing it each time the function is called.